Read The Latest Blog
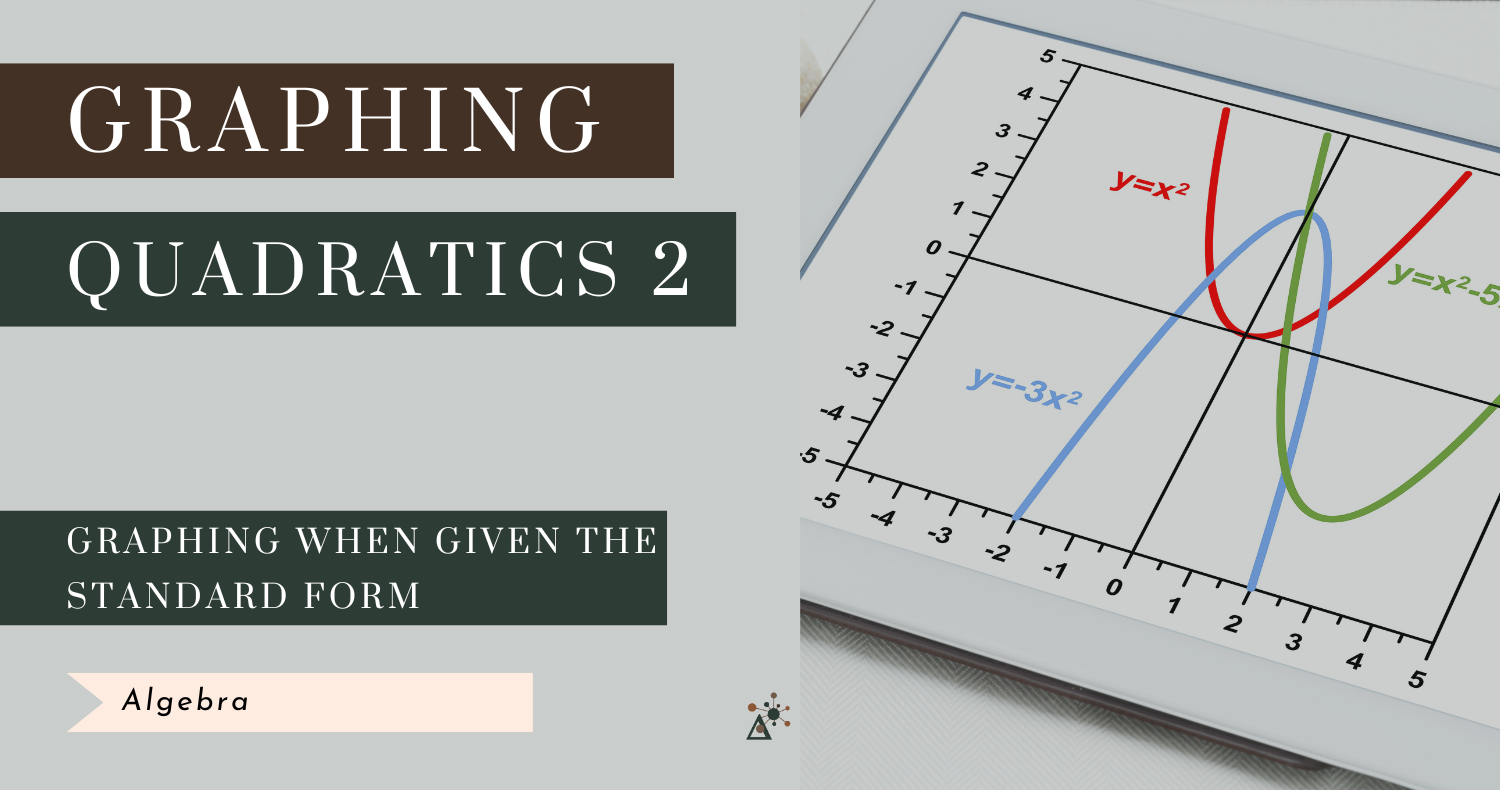
Graphing Quadratic Functions - Standard Form
Learn how to graph quadratic functions in standard form. Discover step-by-step methods to find the vertex, y-intercept, and plot additional points using symmetry. Perfect for mastering parabolas and enhancing graphing skills!
Understanding the Properties of Operations
This blog post explores the essential properties of operations in mathematics, including closure, commutative, associative, distributive, identity, inverse, and equality properties. It provides clear definitions and examples for each property, highlighting how they apply to real numbers and help in understanding algebraic concepts. The post also covers additional properties like the reflexive and zero multiplicative properties, emphasizing their importance in solving mathematical problems. Perfect for students or educators, this post offers a solid foundation for mastering the rules governing basic mathematical operations.

Graphing Quadratics (Vertex Form)
Explore how to graph quadratics in vertex form. This article provides a detailed guide to understanding and graphing quadratic functions. It begins by introducing the basic structure of quadratic functions and the importance of the parent function f(x) = x^2, highlighting the key characteristics like the U-shaped parabola, symmetry, and the vertex. The post then focuses on how to graph quadratics using vertex form f(x) = a(x-h)^2 + k, explaining how the parameters a, h, and k affect the graph. It discusses vertical stretches, compressions, reflections, and both horizontal and vertical shifts. The post concludes with step-by-step instructions on how to graph a quadratic function and provides practice problems for reinforcing the concepts.
Solving a Function for a Variable
In our latest blog post, students will explore the essence of solving functions by learning how to find a variable when given an output. Through algebraic, graphical, and tabular methods, students will uncover diverse strategies to tackle function-solving challenges. This comprehensive guide aims to enhance problem-solving skills, clarify concepts, and empower students with versatile techniques for unraveling the complexities of functions. Join us on this educational journey to master the art of function-solving and confidently approach mathematical tasks with precision and confidence.

Evaluating Functions - When Input Is an Expression
Ready to explore more advanced evaluations? Dive into the second part of our series on function evaluations. Here, the focus is on evaluating expressions as function inputs. Discover techniques for simplifying expressions and substituting them as inputs, and gain step-by-step instructions for evaluating functions algebraically. With this comprehensive guide, you'll gain the skills to confidently evaluate functions with any input expression.

